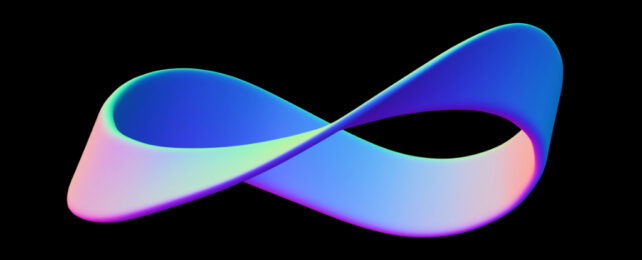
For nearly fifty years, mathematicians have puzzled over a deceptively simple question: how small can you make a Möbius strip without it intersecting itself?
Now, Richard Schwartz, a mathematician at Brown University, has proposed an elegant solution to this problem, which was originally posed by mathematicians Charles Weaver and Benjamin Halpern in 1977.
In their paper, Halpern and Weaver pose a limit for Möbius strips based on the familiar geometry of folded bits of solid paper – that the ratio between the length and width of the paper must be greater than √3, or around 1.73.
For example, a Möbius strip one centimeter in length would need to be wider than √3 or 1.73 centimeters.
Schwartz says he became "hooked" on the Möbius strip problem after learning about it four years ago during a conversation with a colleague.
He had several stabs at solving it over the years and published a paper in 2021 with a promising approach that ultimately fell short.
Schwartz couldn't leave the problem alone and recently started experimenting with squashing paper Möbius strips in the hope that the 2D shape would be easier to tackle mathematically.
But when he cut open one of these loops at an angle (which was necessary to solve his optimization problem), he saw something he did not expect.
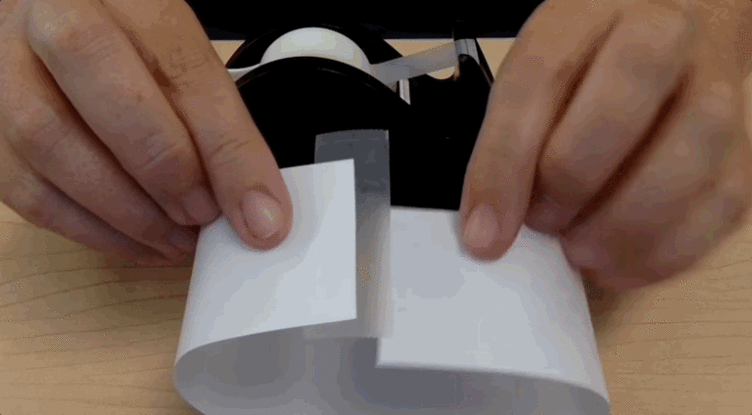
The 2D length of paper didn't look like a parallelogram, as he had reported in his first paper. Rather, it was a trapezoid – a shape with four, straight sides, where only two of the sides are parallel to one another.
"Embarrassingly, I discovered recently that I made an error in setting up the optimization problem," Schwartz writes.
Over three sleepless nights – and with some help from a few colleagues – Schwartz corrected his error and found "a really nice proof" for the intermediate step "that greatly simplified" the paper.
"I was amazed and delighted to discover that when I did the optimization problem correctly, I got … √3 right on the nose!" he writes.
Möbius strips have many strange properties, which has made them objects of fascination since they were described in 1858 by German mathematicians August Möbius and Johann Listing.
Möbius strips are unorientable. This means that an ant wandering around a Möbius strip is never truly on the 'inside' or 'outside' or 'top' or 'bottom' of the shape.

On their travels, the ants cover both sides of the ribbon in one continuous movement.
This ability to use both sides of a surface without the need to flip the ribbon has made Möbius strips useful for tape recorders, typewriters, conveyor belts, print cartridges and roller coasters.
Möbius strips are used in jewelry, the international symbol for recycling and in the Google drive logo because they are never-ending loops.

This paper was made available as a preprint through arXiv.